Original post from 2017
Order of operations used to get right on my wick. I used to hate teaching it.
Mainly because I did it really badly. Just by showing it and going ‘but it’s a convention! It’s needed for other stuff!’
The problem with the order of operations isn’t the operations themselves, but it’s in their reading of the maths in the first place. Naturally, students read from left to right, but really, those more experienced will look at the problem as a whole and prioritise their attention to the bits they’re going to do first.
I liken this to looking at a photograph. We don’t look at the top left corner of an image and examine each pixel to the top right, repeating for each row.
I demonstrate this with a photograph of Mont Blanc.

The Largest Photograph In The World
The about section is fascinating as well, and well worth pointing students in that direction for those so inclined.
Zooming right in on a bit of the sky in the full screen version of the image, I slowly scroll right for a bit until I’ve made my point, then go back, nudge down, and start scrolling right again as if I were ‘reading’ the photograph like I would read a text.

This is clearly a daft way to look at a picture, so we have to zoom out for the full effect.
We notice the mountains first. We look at the big stuff for the overall feel of what’s going on and then we look for more detail, such as the little cable car.
This is how we ‘read’ maths. Big stuff first, then details.
Previously I’ve used GEMA as a reformed BIDMASer, but currently work in a department where a pyramid is dept. policy.
Grouping
Exponent
Multiplicative
Additive
The groupings of either brackets or fraction lines etc. serve as highlighters in my increasingly tenuous mixed metaphor. (Or like a little man on the mountain with a big sign going ‘Oi look at me’ dragging your focus to him first.)
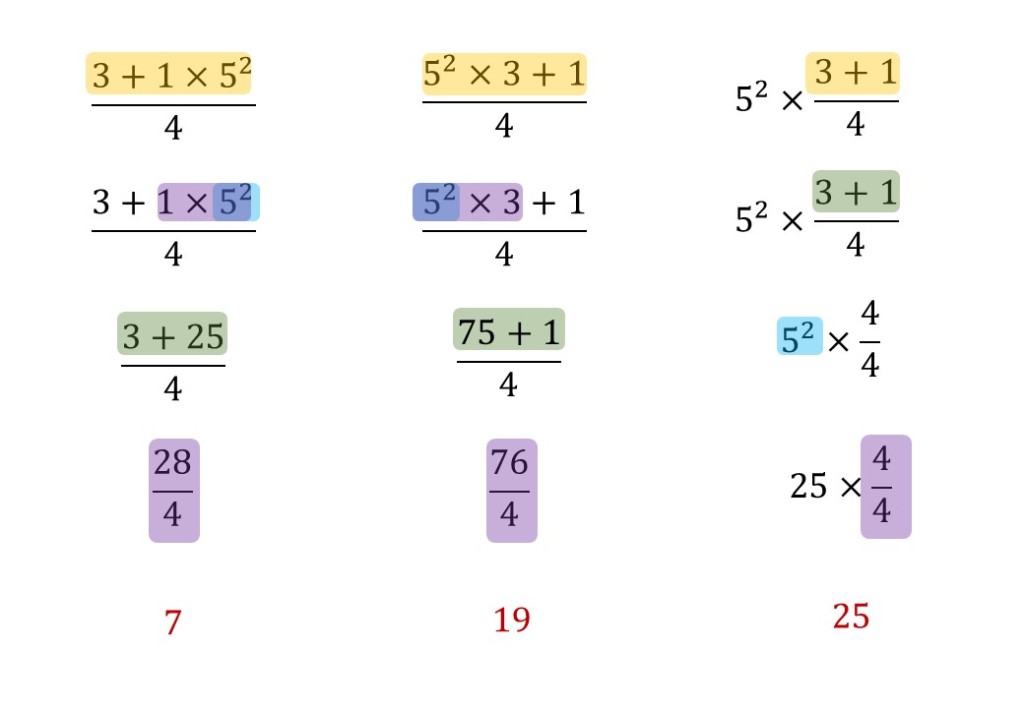
I show the following calculations, starting from the bottom:
33
3×3
3+3
We have addition, then repeated addition, then repeated multiplication, all with two threes. These are all linked by an operation, giving the values 27, 9 and 3 respectively, so in order of size we get the EMA in GEMA. This is contingent on students recognising the links between multiplication and division, and addition and subtraction.
Even then, this seems a bit flimsy when we consider mixtures of operations, for example two threes related in division has a lower value than two threes related by addition. Likewise if we had numbers less than one, the order of size could be different, and that’s without introducing more complex functions. (If you took inverses of the three examples used above though we’d still get the cube root of three > three divided by three > three subtract three.)
The three examples above aren’t meant to be a catch-all explanation of what’s happening, but a way in for students to gain familiarity with the convention.
Students also become more familiar with reading mathematics, and its difference to reading language, which is a valuable habit for geometry, proof and general revision later on in their maths learning.
I later give examples of how I would answer something more complex like the example below, dealing with negatives, a fraction line and a vinculum. This also starts to embed doing something with the quadratic formula instead of painstakingly transcribing it into a calculator, which makes me dry heave.
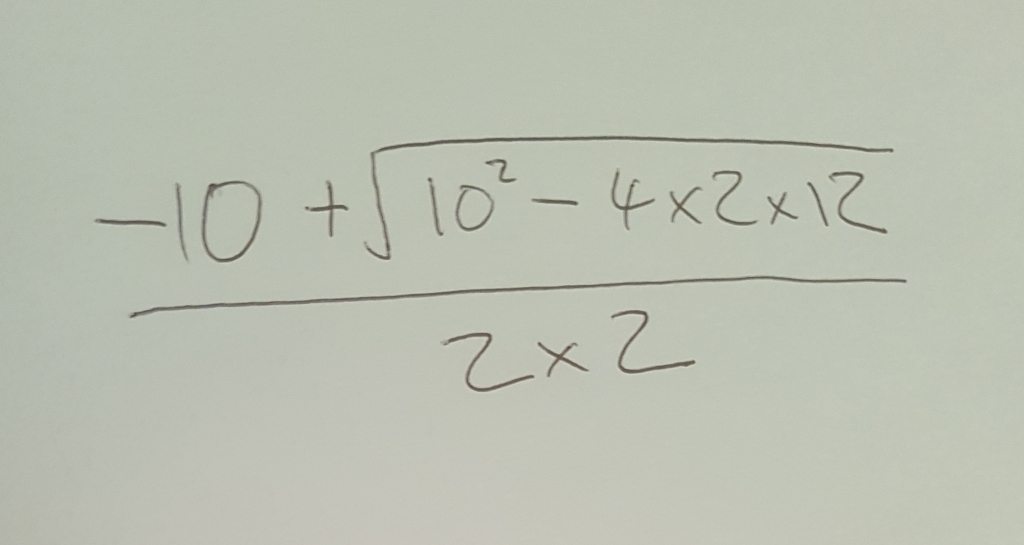
The aim with order of operations is to build up to procedural fluency, as it isn’t a mathematical concept, but a part of mathematical literacy.
Without a thorough grounding in the order of operations, students are going to find it gets in the way of doing maths, much like negative numbers and the foundations of algebra.
I don’t know if this is the optimal way to introduce the order of operations, as it depends on a lot of prior knowledge about the nature of operations. I’m also wary of introducing more metaphor when maths is heavily abstract as it is, but I have found this way of introducing it to be effective.
2021 Update
I deleted very little of the post above coming back to it over four years later.
The reason I’ve come back to it is to add in some resources I’ve made that explicitly move the ideas discussed onward.
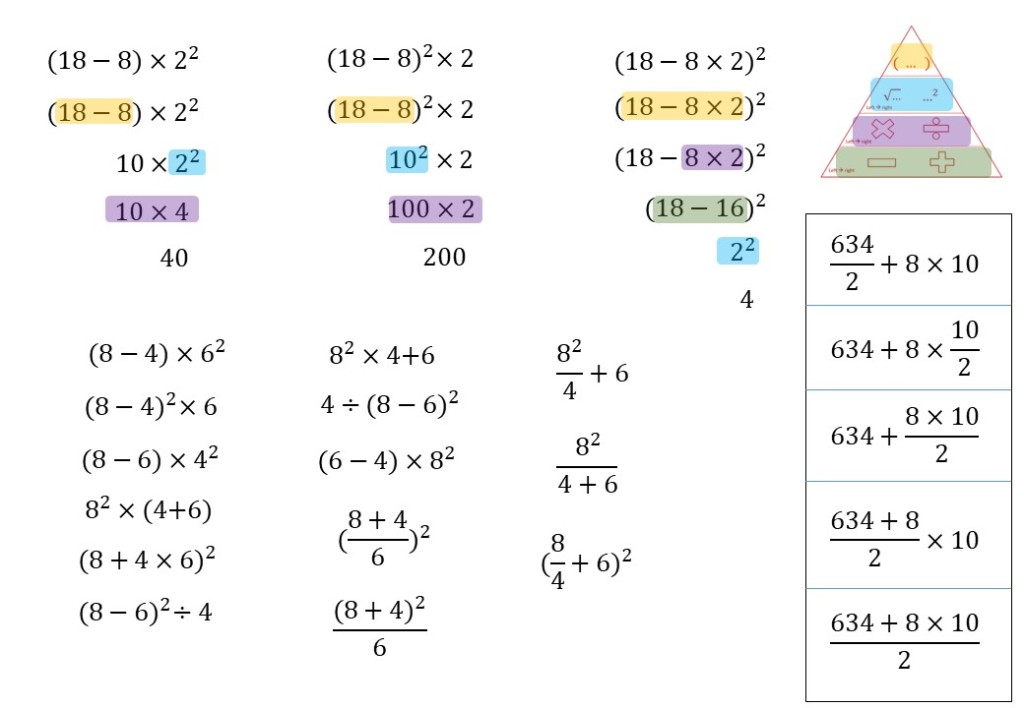
The task below is animated in the slides found at the bottom of the post with colour-coded examples which link to the operation pyramid. These examples and subsequent questions are based around principles of variation.
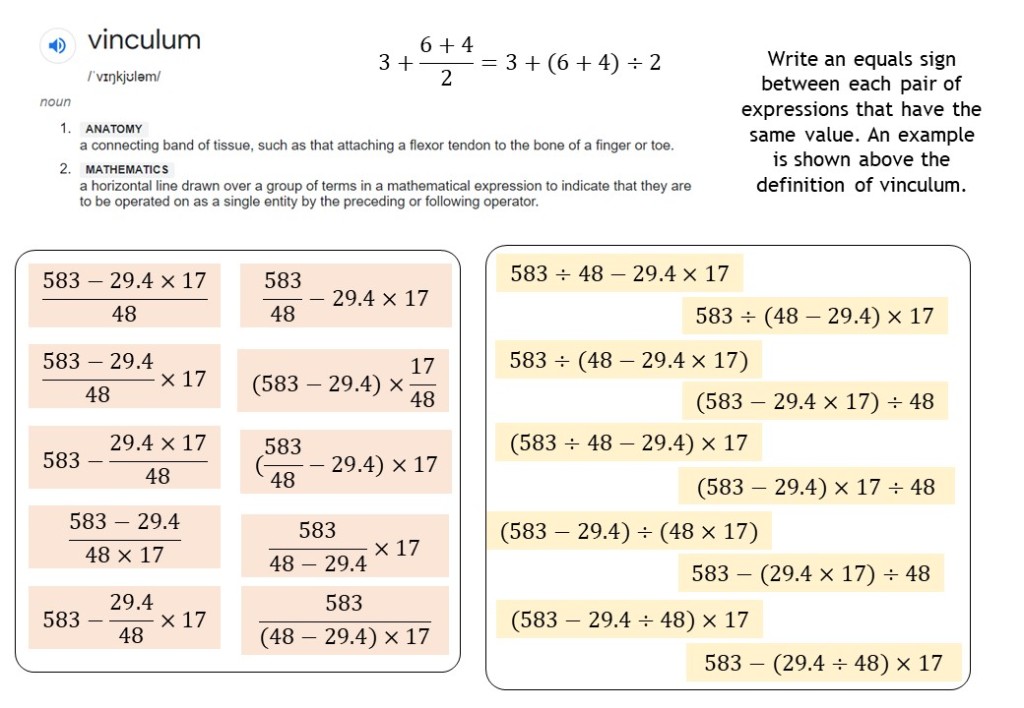
The next set of examples deal with the grouping symbols as a fraction line.
The associated task has questions that all contain five 3s.
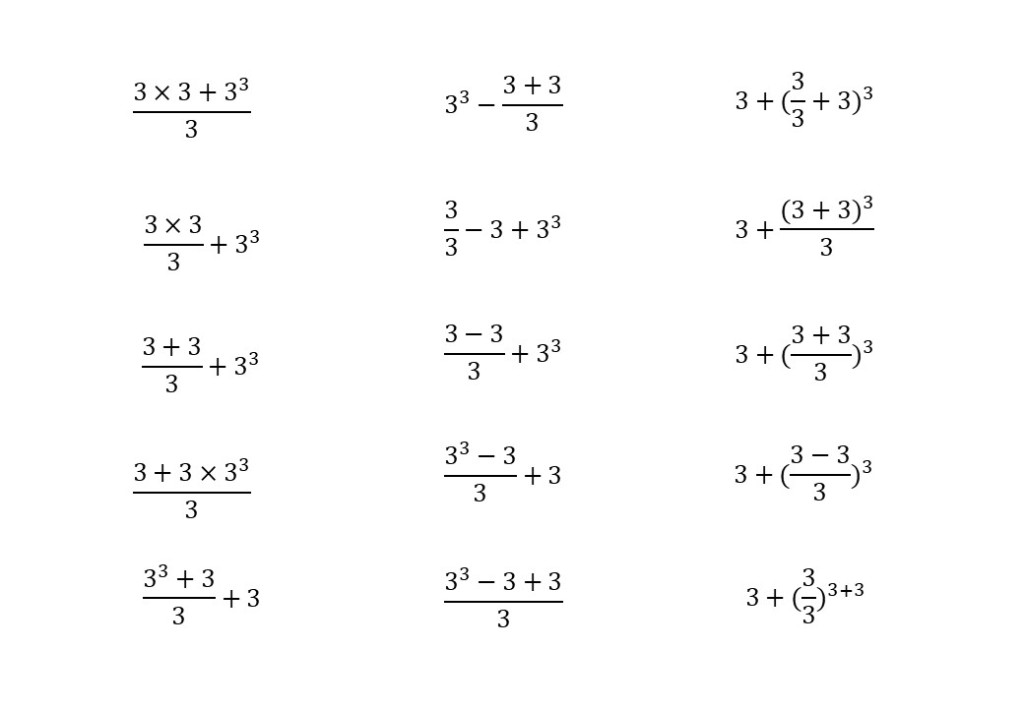
The next task and associated explanation combines the bracket grouping structure representation and fraction line groupings.
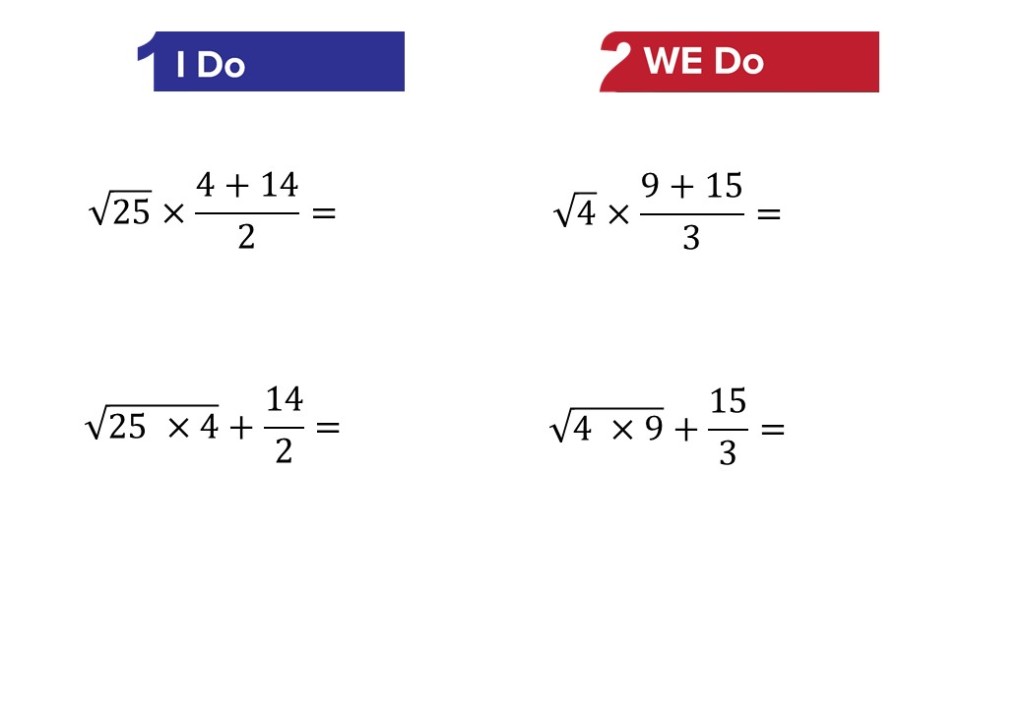
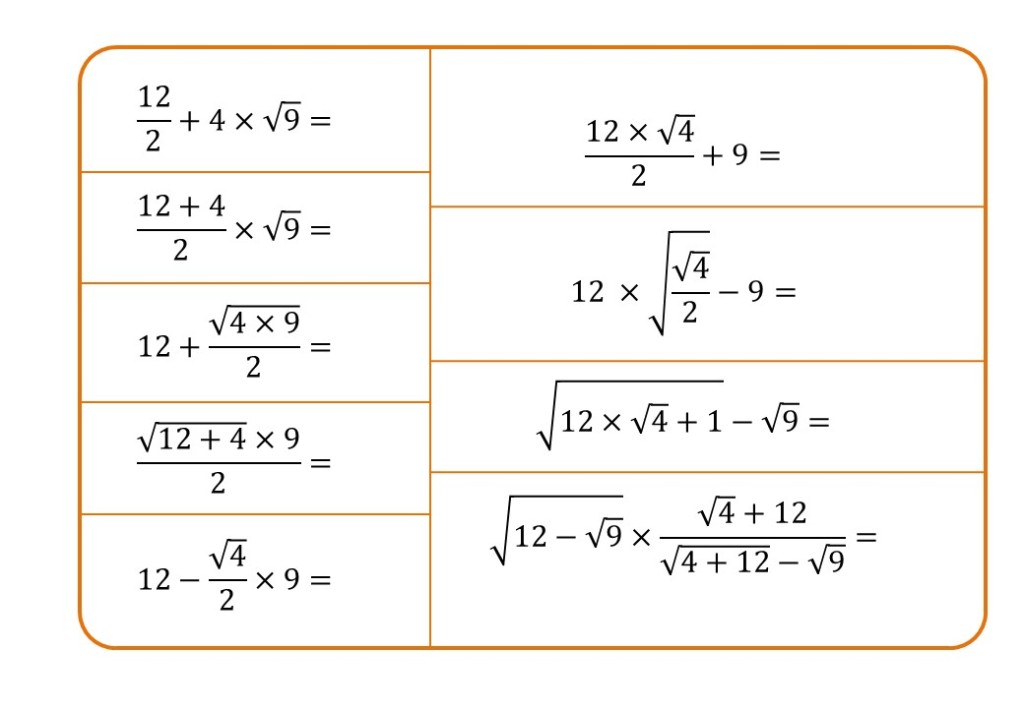
Extending this idea to the vinculum on a square root, the slide below is animated in the slides at the bottom of the post.

The following two slides are adapted from Kyle Gillies’ tasks on variationtheory.com (found here).

I like the idea of GEMA, as it encapsulates what I teach anyway. Pupils who struggle with order of operations are, as you suggest, going to be those for whom the implicit relationships between multiplication & division and addition & subtraction are not fully understood or appreciated. By grouping them within the mnemonic it could help to reinforce these concepts. I always emphasise the point that BIMDSA is perfectly correct but a rubbish mnemonic!
I think I would still teach BIDMAS in the earlier years partly because it does the job satisfactorily as well as it being a fairly ubiquitous concept so they will come across it with other teachers and possibly in other subjects especially Physics. On revisiting the topic especially as algebra gets harder would then be a good time to teach GEMA. They will then be dealing with larger fractions, brackets and roots so will have a sensible range of G and E elements to have to tackle to justify and indeed support the use of the new mnemonic.
LikeLike
I understand what you mean. I try and go all in on the links between mult/div and add/sub v early with my classes so it’s not that much of a barrier for them. (“If you know 4×3=12, you also know 3×4, 12/3 and 12/4. What shop gives a buy one get three free?!?!” Etc.) I’m quite weary of teaching more than one mnemonic for the same process as it gets more complicated as maths is already at a level of abstraction students can find difficult to access. You’ve given me lots to think about Mr H! 🙂 Thank you for taking the time to comment.
LikeLike